15

Access Answers of Maths NCERT Class 9 Chapter 15 – Probability

Exercise 15.1 Page: 283

Q1. In a cricket match, a batswoman hits a boundary 6 times out of 30 balls she plays. Find the probability that she did not hit a boundary.

Sol: According to the question,
Total number of balls = 30
Numbers of boundary = 6
Number of time batswoman didn’t hit boundary = 30 – 6 = 24
Probability she did not hit a boundary = 2430 = 1=45

Q2. 1500 families with 2 children were selected randomly, and the following data were recorded:

Number of girls in a family	2	1	0
Number of families	475	814	211

Compute the probability of a family, chosen at random, having
(i) 2 girls                (ii) 1 girl                   (iii) No girl
Also check whether the sum of these probabilities is 1.

Q3. Refer to Example 5, Section 14.4, Chapter 14. Find the probability that a student of the class was born in August.
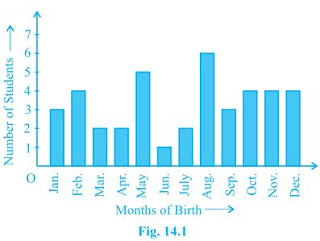
Solution:

Total numbers of students in the class = 40
Numbers of students born in August = 6
The probability that a student of the class was born in August, = 640 = 320

Q4. Three coins are tossed simultaneously 200 times with the following frequencies of different outcomes:

Outcome	3 heads	2 heads	1 head	No head
Frequency	23	72	77	28

If the three coins are simultaneously tossed again, compute the probability of 2 heads coming up.

Sol: Number of times 2 heads come up = 72
Total number of times the coins were tossed = 200
the probability of 2 heads coming up = 72200 = 925

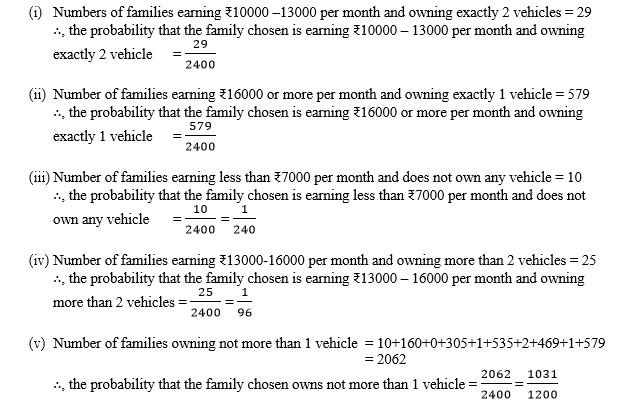
Q5. An organisation selected 2400 families at random and surveyed them to determine a relationship between income level and the number of vehicles in a family. The information gathered is listed in the table below:

Monthly income (in ₹)	Vehicles per family
	0	1	2	Above 2
Less than 7000	10	160	25	0
7000-10000	0	305	27	2
10000-13000	1	535	29	1
13000-16000	2	469	59	25
16000 or more	1	579	82	88

Suppose a family is chosen. Find the probability that the family chosen is
Q earning ₹10000 – 13000 per month and owning exactly 2 vehicles.

• earning ₹16000 or more per month and owning exactly 1 vehicle.
• earning less than ₹7000 per month and does not own any vehicle.
• earning ₹13000 – 16000 per month and owning more than 2 vehicles.
• owning not more than 1 vehicle. 

Sol: Total number of families = 2400

Q6. Refer to Table 14.7, Chapter 14.
(i) Find the probability that a student obtained less than 20% in the mathematics test.
(ii) Find the probability that a student obtained marks 60 or above.Solution:

Marks	Number of students
0 – 20	7
20 – 30	10
30 – 40	10
40 – 50	20
50 – 60	20
60 – 70	15
70 – above	8
Total	90

Total number of students = 90

(i) Number of students who obtained less than 20% in the mathematics test = 7
the probability that a student obtained less than 20% in the mathematics test = 790

(ii) Number of students who obtained marks 60 or above = 15+8 = 23 the probability that a student obtained marks 60 or above =23/90

7. To know the opinion of the students about the subject statistics, a survey of 200 students was conducted. The data is recorded in the following table.

Opinion	Number of students
like	135
dislike	65

Find the probability that a student chosen at random (i) likes statistics, (ii) does not like it.

Sol: Total number of students = 135 + 65 = 200
(i) Number of students who like statistics = 135
the probability that a student likes statistics = 135200 = 2740

(ii) Number of students who do not like statistics = 65
the probability that a student does not like statistics = 65/200 = 1340

Q8. Refer to Q.2, Exercise 14.2. What is the empirical probability that an engineer lives:
(i) less than 7 km from her place of work?
(ii) more than or equal to 7 km from her place of work?
(iii) within km from her place of work?

Sol: The distance (in km) of 40 engineers from their residence to their place of work were found as follows:
5     3     10     20     25     11     13     7     12     31     19     10     12     17     18      11     3      2 17    16     2     7     9     7     8      3     5     12     15     18     3    12    14     2     9     6 15     15     7     6     12

Total numbers of engineers = 40
(i) Number of engineers living less than 7 km from their place of work = 9
the probability that an engineer lives less than 7 km from her place of work =9/40

(ii) Number of engineers living more than or equal to 7 km from their place of work
= 40 – 9 = 31

probability that an engineer lives more than or equal to 7 km from her place of work
= 3140

(iii) Number of engineers living within 12 km from their place of work = 0
the probability that an engineer lives within 1/2km from her place of work = 0/40
= 0

Q9. Activity : Note the frequency of two-wheelers, three-wheelers and four-wheelers going past during a time interval, in front of your school gate. Find the probability that any one vehicle out of the total vehicles you have observed is a two-wheeler.
Sol: The question is an activity to be performed by the students. Hence, perform the activity by yourself and note down your inference.

Q10. Activity : Ask all the students in your class to write a 3-digit number. Choose any student from the room at random. What is the probability that the number written by her/him is divisible by 3? Remember that a number is divisible by 3, if the sum of its digits is divisible by 3.
Sol: The question is an activity to be performed by the students. Hence, perform the activity by yourself and note down your inference.

Q11. Eleven bags of wheat flour, each marked 5 kg, actually contained the following weights of flour (in kg):

4.97      5.05      5.08     5.03     5.00     5.06     5.08      4.98       5.04       5.07       5.00

Find the probability that any of these bags chosen at random contains more than 5 kg of flour.
Sol: Total number of bags present = 11
Number of bags containing more than 5 kg of flour = 7
the probability that any of the bags chosen at random contains more than 5 kg of flour
=711

Q12. In Q.5, Exercise 14.2, you were asked to prepare a frequency distribution table, regarding the concentration of sulphur dioxide in the air in parts per million of a certain city for 30 days. Using this table, find the probability of the concentration of sulphur dioxide in the interval 0.12-0.16 on any of these days.

The data obtained for 30 days is as follows:
0.03      0.08      0.08      0.09      0.04      0.17      0.16      0.05      0.02      0.06      0.18      0.20      0.11      0.08      0.12      0.13      0.22      0.07      0.08      0.01      0.10      0.06      0.09      0.18      0.11      0.07      0.05      0.07      0.01      0.04
Sol: Total number of days in which the data was recorded = 30 days Numbers of days in which sulphur dioxide was present in between the interval 0.12-0.16 = 2 the probability of the concentration of sulphur dioxide in the interval 0.12-0.16 on any of these days = 230 = 115

Q13. In Q.1, Exercise 14.2, you were asked to prepare a frequency distribution table regarding the blood groups of 30 students of a class. Use this table to determine the probability that a student of this class, selected at random, has blood group AB.
The blood groups of 30 students of Class VIII are recorded as follows:
A, B, O, O, AB, O, A, O, B, A, O, B, A, O, O, A, AB, O, A, A, O, O, AB, B, A, O, B, A, B, O.

Sol: Total numbers of students = 30 Number of students having blood group AB = 3 the probability that a student of this class, selected at random, has blood group AB = 330 = 110